Friday, 22 February 2013
Description of Language
TANAKA Akio
September 9, 2011
September 9, 2011
<Preparation>
Manifold M
Cup product map of M 
Dual map of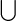 
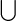
Free Lie algebra that 
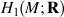generates £ ( 
)
generates £ ( )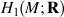
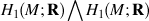is identified as the partial space of £( 
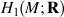) that quadrastic Lie bracket of 
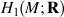generates.Ideal of £(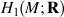 
) that Im η generates a
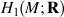) that Im η generates a Holonomy Lie algebra of M 
Completion of holonomy Lie algebra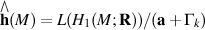 
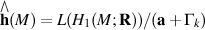
<Theorem>
If M has quadrastic homology connection, Malcev completion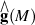
becomes isomorphic with holonoly Lie algebra completion 
.
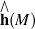If M has quadrastic homology connection, Malcev completion
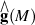becomes isomorphic with holonoly Lie algebra completion 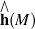.<Theorem>
Primary minimum model M(1)of differential manifold M is isomorphic with Malcev completion of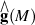
of M's fundamental group.
Primary minimum model M(1)of differential manifold M is isomorphic with Malcev completion of
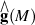of M's fundamental group.<Interpretation>
For the description of a language model there is a need primary minimum model M(1) of differential manifold M.
© 2011 by The Sekinan Research Field of Language
No comments:
Post a Comment