Connection of Words
0.
[Preface]
From Distance to Pseudo-Kobayashi-Distance
[Preface]
From Distance to Pseudo-Kobayashi-Distance
1.
C is complex plane.
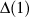 is unit disk which center is the origin of C.
is unit disk which center is the origin of C.
z, w are the two points of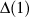 .Hyperbolic distance
.Hyperbolic distance 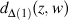 between z and w are defined by the next.
between z and w are defined by the next.
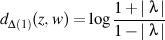 ,
, 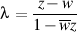 .
.
C is complex plane.
 is unit disk which center is the origin of C.
is unit disk which center is the origin of C.z, w are the two points of
 .Hyperbolic distance
.Hyperbolic distance  between z and w are defined by the next.
between z and w are defined by the next.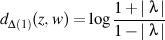 ,
, 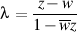 .
.2.
M is complex manifold.
x, y are arbitrary points of M.
fv is finite sequence of regular curve.
Point zv is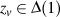 .
. ,
, 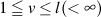 .
.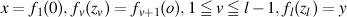 .{
.{ 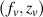 } is called regular chain.Kobayashi pseudodistance dM is defined by the next.
} is called regular chain.Kobayashi pseudodistance dM is defined by the next.
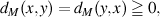
 .
.
M is complex manifold.
x, y are arbitrary points of M.
fv is finite sequence of regular curve.
Point zv is
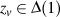 .
. ,
, 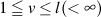 .
.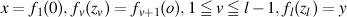 .{
.{ 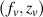 } is called regular chain.Kobayashi pseudodistance dM is defined by the next.
} is called regular chain.Kobayashi pseudodistance dM is defined by the next.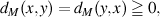
 .
.3.
[Interpretation on 2.]
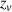 := Meaning minimum of word.dM := Distance of word.
:= Meaning minimum of word.dM := Distance of word.
M:= Word.
[Interpretation on 2.]
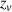 := Meaning minimum of word.dM := Distance of word.
:= Meaning minimum of word.dM := Distance of word.M:= Word.
4.
[Definition]
When dM becomes distance function, M is called Kobayashi hyperbolic.
When dM becomes complete distance, M is called complete Kobayashi hyperbolic.
[Definition]
When dM becomes distance function, M is called Kobayashi hyperbolic.
When dM becomes complete distance, M is called complete Kobayashi hyperbolic.
5.
When M = is satisfied at dM , dM is equal to Poincaré distance.
is satisfied at dM , dM is equal to Poincaré distance.
When M =
 is satisfied at dM , dM is equal to Poincaré distance.
is satisfied at dM , dM is equal to Poincaré distance.6.
X is complex manifold.
M is contained in X as relative compact.
7.
[Definition]
What embedding is hyperbolic embedding is defined by the next.M is KObayashi hyperbolic.
is hyperbolic embedding is defined by the next.M is KObayashi hyperbolic.
Arbitrary boundary points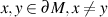 .
.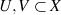 .
.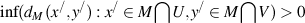 .
.
X is complex manifold.
M is contained in X as relative compact.
7.
[Definition]
What embedding
 is hyperbolic embedding is defined by the next.M is KObayashi hyperbolic.
is hyperbolic embedding is defined by the next.M is KObayashi hyperbolic.Arbitrary boundary points
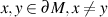 .
.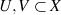 .
.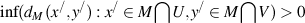 .
.8.
[Theorem,Kwack 1969]
When M is hyperbolicly embedding in X,
What arbitrary regular map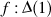 \{0}
\{0}  is regularly connected to
is regularly connected to 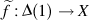 .
.
[Theorem,Kwack 1969]
When M is hyperbolicly embedding in X,
What arbitrary regular map
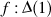 \{0}
\{0}  is regularly connected to
is regularly connected to 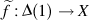 .
.9.
[Interpretation on 6,7,8,9]
X:= Language.
M:= Word.
 := Distance of word.
:= Distance of word.
 := Connection of words.
:= Connection of words.
[Interpretation on 6,7,8,9]
X:= Language.
M:= Word.
 := Distance of word.
:= Distance of word. := Connection of words.
:= Connection of words.10.
[Conjecture, Kobayashi]
(i) If d is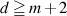 , degree d's general hypersurface X of
, degree d's general hypersurface X of 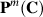 is Kobayashi hyperbolic.(ii)If d is
is Kobayashi hyperbolic.(ii)If d is 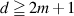 ,
, 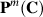 \
\ 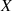 is hyperbolicly embedded in
is hyperbolicly embedded in 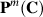 .
.
[Conjecture, Kobayashi]
(i) If d is
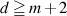 , degree d's general hypersurface X of
, degree d's general hypersurface X of 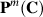 is Kobayashi hyperbolic.(ii)If d is
is Kobayashi hyperbolic.(ii)If d is 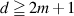 ,
, 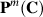 \
\ 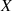 is hyperbolicly embedded in
is hyperbolicly embedded in 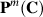 .
.11.
[Interpretation on 10.]
X:= Language.
d:= Hierarchy of language.
[Interpretation on 10.]
X:= Language.
d:= Hierarchy of language.
12.
[References]
<On meaning minimum of word>
From Cell to Manifold / Cell Theory / Tokyo June 2, 2007
Amplitude of Meaning Minimum / Complex Manifold Deformation Theory / Tokyo December 17, 2008
<On distance of word>
Distance Theory /Tokyo May 5, 2004
Distance of Word / Complex Manifold Deformation Theory / Tokyo November 30, 2008
<On connection of words>
Quantum Theory for Language / Tokyo January 15, 2004
<On hyperbolicity>
Reflection of Word / Complex Manifold Deformation Theory / Tokyo December 7, 2008
Boundary of Words / Topological Group language Theory / Tokyo February 12, 2009
[References]
<On meaning minimum of word>
From Cell to Manifold / Cell Theory / Tokyo June 2, 2007
Amplitude of Meaning Minimum / Complex Manifold Deformation Theory / Tokyo December 17, 2008
<On distance of word>
Distance Theory /Tokyo May 5, 2004
Distance of Word / Complex Manifold Deformation Theory / Tokyo November 30, 2008
<On connection of words>
Quantum Theory for Language / Tokyo January 15, 2004
<On hyperbolicity>
Reflection of Word / Complex Manifold Deformation Theory / Tokyo December 7, 2008
Boundary of Words / Topological Group language Theory / Tokyo February 12, 2009
13
[History]
Distance of language Historical Review
[History]
Distance of language Historical Review
14.
[Appendix]
The Time of Language / Tokyo January 10, 2012
[Appendix]
The Time of Language / Tokyo January 10, 2012
Tokyo
February 3, 2012
April 7, 2020 Text errata partly corrected
Sekinan Research Field of Language
February 3, 2012
April 7, 2020 Text errata partly corrected
Sekinan Research Field of Language

No comments:
Post a Comment